About the CoMSES Model Library more info
Our mission is to help computational modelers at all levels engage in the establishment and adoption of community standards and good practices for developing and sharing computational models. Model authors can freely publish their model source code in the Computational Model Library alongside narrative documentation, open science metadata, and other emerging open science norms that facilitate software citation, reproducibility, interoperability, and reuse. Model authors can also request peer review of their computational models to receive a DOI.
All users of models published in the library must cite model authors when they use and benefit from their code.
Please check out our model publishing tutorial and contact us if you have any questions or concerns about publishing your model(s) in the Computational Model Library.
We also maintain a curated database of over 7500 publications of agent-based and individual based models with additional detailed metadata on availability of code and bibliometric information on the landscape of ABM/IBM publications that we welcome you to explore.
Displaying 3 of 3 results negative feedback clear search
External shocks, agent interactions, and endogenous feedbacks--investigating system resilience with a stylized land use model
Yang Chen | Published Tuesday, March 06, 2018The purpose of the presented ABM is to explore how system resilience is affected by external disturbances and internal dynamics by using the stylized model of an agricultural land use system.
We explore land system resilience with a stylized land use model in which agents’ land use activities are affected by external shocks, agent interactions, and endogenous feedbacks. External shocks are designed as yield loss in crops, which is ubiquitous in almost every land use system where perturbations can occur due to e.g. extreme weather conditions or diseases. Agent interactions are designed as the transfer of buffer capacity from farmers who can and are willing to provide help to other farmers within their social network. For endogenous feedbacks, we consider land use as an economic activity which is regulated by markets — an increase in crop production results in lower price (a negative feedback) and an agglomeration of a land use results in lower production costs for the land use type (a positive feedback).
How to not get stuck – an ant model showing how negative feedback due to crowding maintains flexibility in ant foraging
Tomer Czaczkes | Published Thursday, December 17, 2015Positive feedback can lead to “trapping” in local optima. Adding a simple negative feedback effect, based on ant behaviour, prevents this trapping
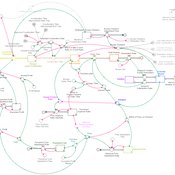
Endogenous Dynamics of Housing Market Cycles
Onur Özgün Birnur Özbaş Yaman Barlas | Published Monday, September 09, 2013 | Last modified Wednesday, January 08, 2014The purpose of this model is to analyze the dynamics of endogenously created oscillations in housing prices using a system dynamics simulation model, built from the perspective of construction companies.